Derivative of the exponential function

In the article what is e?, we stated that:

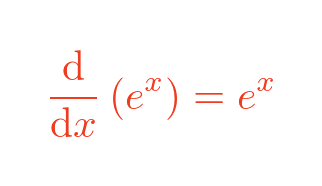
This is an important result. It tells us that the exponential function is its own derivative. This means that we can use it to solve equations where the rate of x change is proportional to the value of x.

In this article, we will prove this result, and look at some of the consequences.

Derivative of the exponential function

To prove the result we will start from first principles. The derivative of a function y = f(x) is given by:

In the case of the exponential function e^x, this gives us:

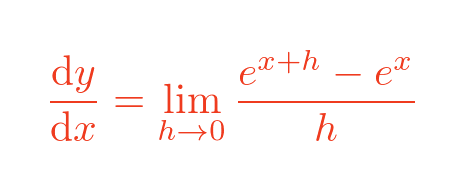
From the rules of exponents we know that e^{x + h} is e^xe^h so we can extract the common factor of e^x:

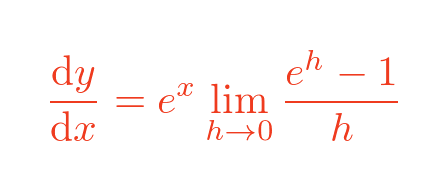
Now let's look at the definition of e we developed in the formula for e article:

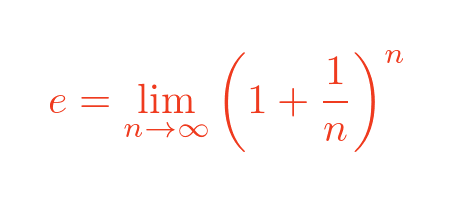
We can substitute the value 1/h = n is this formula:

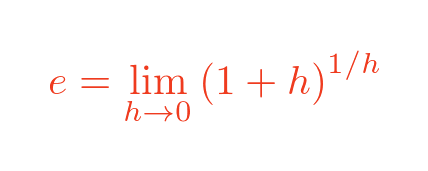
Notice that we have changed the limit. The equation in n applies as n tends to infinity. And n tends to infinity, h = 1/n tends to zero.

We can substitute this value for e into the main equation:

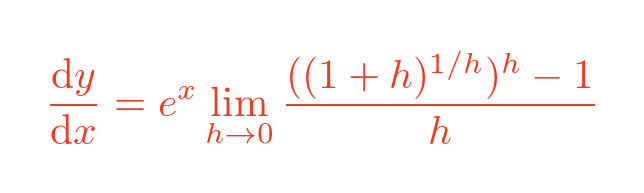
Again from the rules of exoponents we know that (e^a)^b is e^ab so we can simplify this term (the power of 1/h and the power of h cancel each other out):

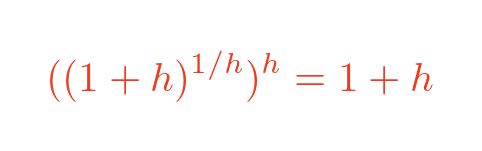
This further simplifies to:

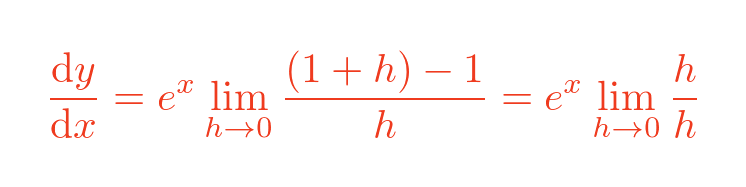
The limit of h/h as h tends to zero is 1, so we get the final result:

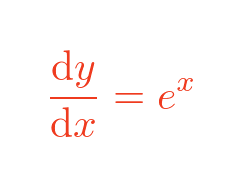
Why e?

You might still be wondering why the magic value of e is involved in this equation. Well if we go back to this equation:

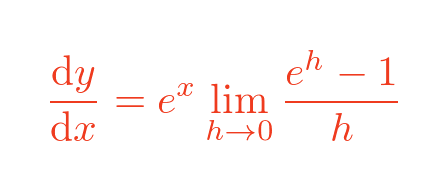
We see that the crucial fact is that the limit term is 1:

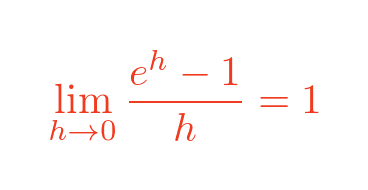
We won't prove it here, but for this limit to be true essentially requires e^x to have a slope of 1 at x = 0.

These graphs show exponential functions with bases of 2, e and 5, with a dotted black line of slope 1:

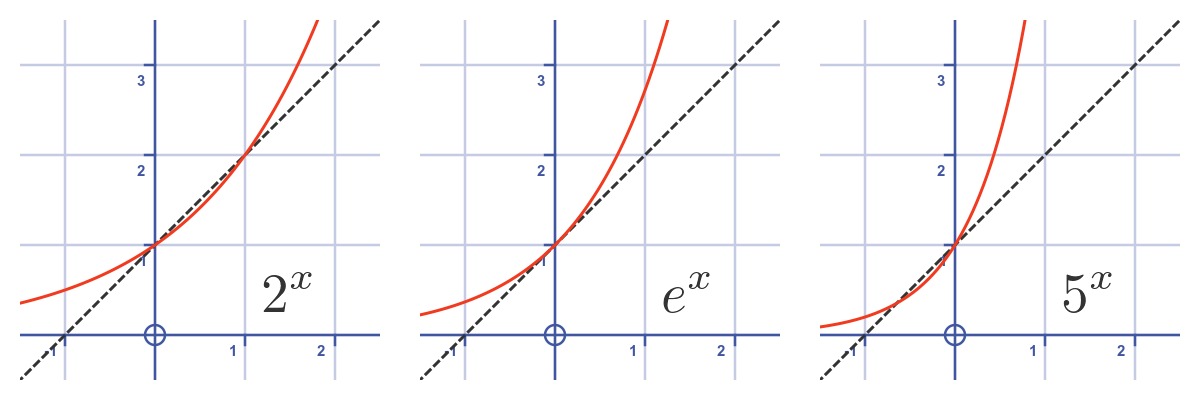
Base e has a slope of 1 when x is zero. Base 2 has a slope of less than 1, base 5 has a slope of greater than 1.

A new definition of e

We can now provide an alternative definition of e:

e is the base value for which the function a to the power x has a slope of 1 when x is zero.

Given this new definition, it is a lot easier to understand why e has such special properties.

But why is this definition valid? If you recall the article formula for e, our starting point was a bank account that paid 100% per year. So £1 turned into £2 in the first year, giving a slope of £1/year.

We deliberately set the slope to 1, and the value of e arose out of that initial condition.

If we draw an exponential function with a slope of 1 at x = 0, that function will have a base of e. This is similar to saying that if you draw a circle of radius 1 it will have an area of pi.

The value e, like pi, is what it is - a strange irrational number that arises out of a simple situation.

Integration

Since the derivative of e^x is e^x, it follows that the integral is also e^x.

More precisely, the indefinite integral of e^x is:

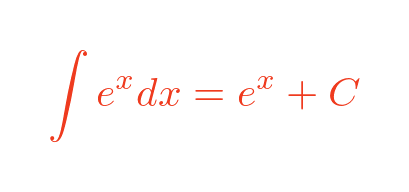
Where C is the constant of integration.

Since the function tends to zero as x tends to minus infinity, we have the following definite integral:

In other words, the area under the curve from a, stretching back to minus infinity, is e to the power a*. This is shown graphically here:

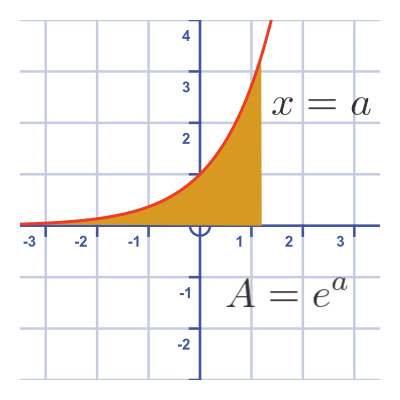
The area A under the curve is equal to the value of the curve

See also

• Rules of exponents
• Negative and fractional exponents
• Graphs of exponential functions
• 2 to the power π - exponential function for an irrational value of x
• What is e?
• Formula for e
• Proof that e is irrational